One of the key challenges in measuring the electrical current in high voltage, high power transmission lines, is that of measurement circuit isolation. One way of measuring heavy 60 Hz currents in a conductor is through the use of a current transformer. A current transformer typically consists of a number of turns of wire wound on a high permeability, toroid magnetic core. The current carrying conductor is passed through the ‘hole’ in the toroid and its magnetic field induces a voltage in the toroid’s winding, which is proportional to the current in the conductor. This induced voltage is measured with an AC millivoltmeter. This means of measuring heavy AC currents works well at lower voltages on the conductor. However, when the voltage on the conductor reaches values too high to safely isolate the toroid’s winding and of course the meter circuit from the conductor, some other ways of measurement must be employed to ensure safety. Magneto-optic current sensors provide a means to overcome this challenge.
The Concept of Magneto-Optic Current Sensors
The Faraday Magneto-Optic Effect provides a way to measure high currents on conductors at extremely high voltages above ground (50 kV and higher), with reasonable accuracy and exceptional isolation. Magneto-Optic Current Sensors (MOCS), which make use of the Faraday magneto-optic effect, use either solid glass pathways or fiber-optic waveguides. When linearly polarized light is passed through a transparent, diamagnetic material through which a magnetic field is also passed in the same direction, there will be, in general, a rotation of the polarization vector of the emergent ray. It can be demonstrated that the polarization rotation angle α is given by:

The rotation, α, is proportional to the line integral of the magnetic field intensity vector, H, along the light propagation path, l, which encloses the magnetic field. V is the Verdet constant for the material used.
Notice that by the Ampere circuital law:

Where I is the current enclosed by one optical path loop. If N loops of a fiber optic are wound on a form so that all turns are aligned and have the same area, the Faraday magneto-optical polarization rotation may be expressed as follows:
α = VNI [equation 1.2]
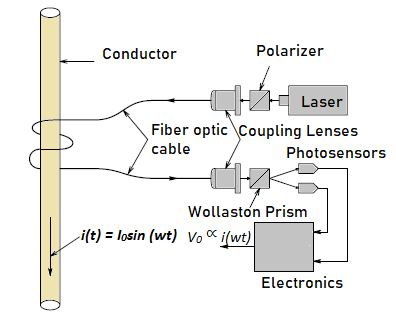
The figure below shows a version of a Faraday current transducer consisting of N turns of single mode optical fiber wound around a current carrying conductor.
Recommended: The Ultimate Guide to Electrical Maintenance

The rotation of the linearly polarized light entering the fiber is given by equation 1.1 above.
Related articles:
- Power Measurement in AC Circuits (Single-phase & Polyphase Systems)
- Power Measurements in DC Circuits
- RF Power and Voltage Measurement
- DC to DC Power Converters: Function, Types, Operation & Applications
- Digital Multimeter: Principle of Operation and How it is used
- How to calibrate a dc ammeter

Leave a Reply
You must be logged in to post a comment.